Can you really measure distance between two points?

Of course! By using a ruler, right?

But in reality, you are coinciding given two points with the points of your ruler. Then you will say, these points are separated by a $x$ unit in length.

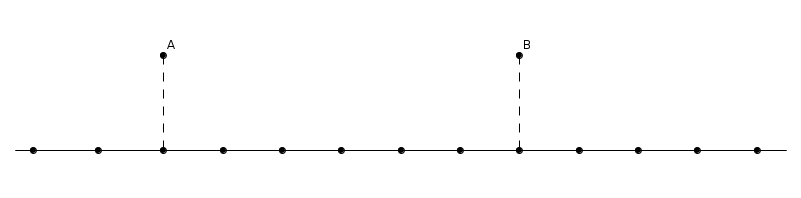
I want to mention now, in the above line consisting of points [see above figure], these points are equally separated. To make an equal separation, you do not need a standard ruler (i.e. with a standard unit). Is that right? This means the line is a ruler but without a unit.

Now, you can count all the points from a coinciding point with A to a coinciding point with B including points lying between A and B in our new ruler then, you will say the distance between A and B is 6 (because in the ruler, counting starts from 0). But, where is its unit? Does 6 mean the distance? No! This 6 means the line coincided 7 points between A and B including themselves. Otherwise, nothing. We can even make small spacing with more points in the line. So, how can we track different spacing and relationships between them? The solution is to assign a “unit” to every spacing. You cannot simply measure spacing with itself. But, you can measure the ratio of the spacing to a standard ruler such that it gives one because of the coinciding concept.

Generally, if one measures a length, one actually measures the ratio of that length to a standard unit of length. i.e.

$ \frac{\text{Distance between AB}}{6 cm \text{ in centimeter ruler}} = 1$

$\therefore \text{Distance between AB} = 6 cm$.

This is how AB gets its unit.

In Quantum Gravity, we are interested to probe the spacetime even below the Planck length (order of $10^{-35}$m) but, we don’t know how to define spacing below that length. In reality, we don’t know does spacetime at that region is discrete or a continuum. But, most of the candidate theory for Quantum Gravity believes that spacetime has a discrete nature, and even length and time have discreteness. So, if the length is discrete how to define a spacing between two points can be a major problem in Quantum Gravity. Because we define spacing AB supposing that it can include infinitely many points between A and B.

Lastly, this is what this article arXiv:1604.01798 trying to explain.